One of the major breakthroughs in condensed matter physics in the 21st century is the discovery of topological insulators. These materials don't conduct electricity in their inner structure, but they do conduct it on their surfaces or edges. The word "topological" comes from topology, a branch of mathematics used to describe the properties of shapes and spaces — in this case, the behavior of electronic structures.
Later, scientists found that similar effects can happen not only with electrons but also with waves like light and sound. This is done using specially designed materials called topological photonic and phononic crystals. In these materials, waves are blocked from moving through the inside of the crystal for certain frequency ranges, called band gaps, but they can still travel along the edges. Waveguides that use this effect are called topological waveguides. They are known to deflect waves less at corners and defects than normal waveguides.
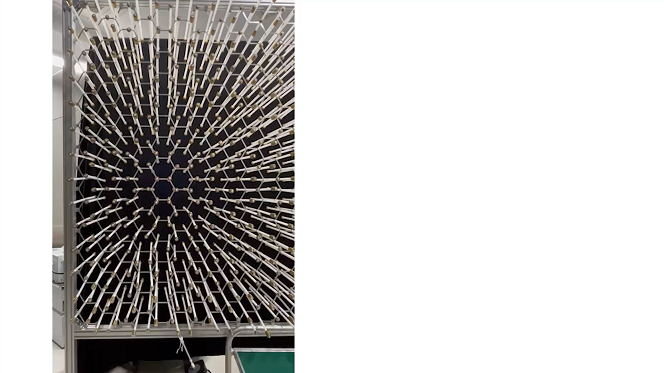
In this study we have built a two-dimensional wave machine to show how topological waveguides work.
In this video, the bar at the bottom is shaken at a steady frequency. At 4.20 Hz on the left hand side, the vibrations spread into the main part of the wave machine. (This frequency is outside a region of forbidden wave propagation called the phononic band gap.) At 4.66 Hz on the right hand side the vibrations move only along the waveguide path, because at this frequency (inside the phononic band gap) the waves are forbidden to spread.
A video showing vibrations moving along the topological waveguide in a two-dimensional wave machine. Click the image to play the animation (3 MB movie).
The original one-dimensional wave machine was made by John Shive at Bell Labs in the United States. Its slow, large motions make it easy to see wave movement with the naked eye, which is why it's often used in physics education around the world. The speed of the twisting waves that move along a thin central wire is reduced by long bars attached to it, making the motion easily visible.
We built a two-dimensional wave machine using a laser cutter to pattern a thin sheet of stainless steel. We then attached rods and weights. By changing where the weights are placed, we created a topological waveguide at the border between two uniform areas.
Topological materials introduce new concepts in physics, such as the Berry phase often also used in quantum mechanics and solid-state physics, and is an interdisciplinary area of study. For more details, see 'Phononic band calculations and experimental imaging of topological boundary modes in a hexagonal flexural wave machine,' H. Takeda, R. Minami, O. Matsuda, O.B. Wright, M. Tomoda, Appl. Phys. Express 17, 017004 (2024).